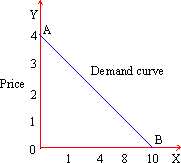
(A) Demand Schedule : The various quantities demanded of a particular commodity are presented here in a schedule. At arbitrarily chosen prices, the quantity of a commodity an individual consumer is expected to demand, is explained by the schedule. Since quantity demanded (q_d) depends on the relevant prices of goods, the two can be expressed in the form of an algebraic function as well. The schedule shows that as price goes on rising (from zero to 4) the quantity demanded goes on falling (from 10 to zero).

The scheduled information has been presented in the form of a demand curve in Figure 2 (below). In the figure, the units of quantity of the goods have been measured along the horizontal axis (OX) and the respective prices have been shown along the vertical axis (OY). The curve intersects OY axis at point A which shows highest price at which quantity demanded is zero. On the contrary the curve intersects OX axis at point B showing largest quantity demanded where price is zero. Both OA and OB are said to be intercept quantities when one of the variables assumes zero value. Note that demand curve is sloping downward. This follows the law of demand (given below). But the demand curve of such a shape is obvious from the fact that quantities demanded and price in the demand schedule hold an inverse relationship.

Quantity Demanded q_d
Figure 2

(B) Demand Function: The price-demand relationship shown above can be expressed in the form of a demand function as follows:

q_d = 10 - 3P

On substitution of any scheduled value of P we get the relevant value of the quantity demanded. Thus when P = 1 then q_d =10 - 3 (1) = 7 or when P = 3, then q_d = 10 - 3 (3) = 1 etc.

(C) Law of demand: The law of demand explains the inverse relation between quantity and price in general. It can be stated as follows:

"Ceteris Paribus (other things remaining equal), the quantity of a good demanded will rise (expand) with every fall in its price and the quantity of a good demanded will fall (contract) with every rise in its price."

In a functional form this can be stated as,

q_d = f (P) [ Y, Ps, N, Z ]_const.

This explains that q_d, the quantity of a good demanded functionally depends on its price P. However, the quantity demanded is also causally related to other factors such as income of an individual (Y), prices of substitutes (Ps), number of members in the family (N) and the tastes of the consumer (Z). In order to satisfy price-demand relation, the effect of these other variables has been restrained by assuming them to be constant.

(A) Supply Schedule: Just as goods are demanded by consumers, they are supplied by manufacturers or sellers. At any point of time quantity supplied by them is a function of the market price. Several such prices can be related to the relevant quantities supplied: this would give the supply schedule. In the given schedule, as price of the goods rises (from zero to 3) the quantity supplied also rises (from zero to 6 units).

Figure 3: Supply

(B) Supply Function: Supply is a direct function of the price and it rises or falls with the price. This is because the law of supply is based on the behavior of the cost of production. Assuming that manufacturers begin at the point where cost of production is minimal any further production and supply of goods can be possible only at an increasing additional or marginal cost per unit. Hence they can afford to supply more only at a rising price. Further, logically any seller would be willing to sell more goods if the price were to rise. The quantity supplied at the given range of prices as above can be presented in the form of an algebraic function:

q_s = 2P

With the help of the function we can find the quantity supplied at any randomly chosen prices. For instance, when P = 3, q_s = 6 or when P = 2, q_s = 4 etc.

Law of Supply: The law of supply can be stated as follows:

"Ceteris paribus, the quantity of a good supplied will rise (expand) with every rise in its price and the quantity of a good supplied will fall (contract) with every fall in its price."

In a functional form this can be stated as :
q_s = f (P) [T, R, P] _const.

The quantity of a commodity supplied is thus a function of its own price. There exists a direct relationship between the quantity supplied and the price of a commodity. It is subject to the condition that other things should remain constant. In this case ‘other things’ include mainly two things. These are technical conditions or methods of production (T) and the prices and quantities of the resources supplied (RP). With improved technical conditions, supply can be increased at the same old price, since the cost of production can now be reduced. Similarly with an enhanced supply of resources and a reduction in the prices of resources such as land, labor, raw materials etc. an increasing quantity of the commodity can be supplied at a constant or even falling price.

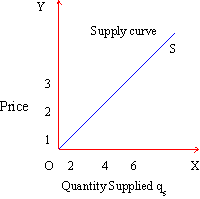
Figure 4

Figure 4 is the graphical representation (the supply curve) of the supply schedule. It begins at the point of origin where both quantity supplied and price are zero in value, and then it continuously rises upwards. This upward sloping curve indicates the positive relationship between supply and price: there is a rise in the quantity supplied with every successive rise in the price.

(D) Expansion or contraction and increase or decrease: Changes in the quantity supplied as a result of movement along the same supply curve has been described by Marshall as rise and fall or expansion and contraction of quantity supplied of the commodity. But if the supply curve shifts left or right of the original curve, the changes in supply of the good are known as increase or decrease.

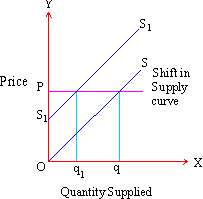
Figure 5

In figure 5 we notice such a shift in the supply curve. On the original supply curve (OS) the quantity of goods supplied at price OP is Oq but when the supply curve shifts towards its left (i.e. S₁ S₁) then at the same price OP, the quantity supplied decreases to Oq₁. If we begin with S₁ S₁ as the original supply curve, OS would represent a shift of the supply curve towards the right. In this case, quantity supplied increases at a given price.

The supply curve undergoes a shift in it with a change in the technical conditions or the price and supply conditions of the inputs (resources). With improved techniques or methods of production, the degree of the efficiency with which some or all resources can be utilized will increase. This results in a favorable change in the cost of production. Similarly with improved supplies and reduced prices of the inputs, the cost of production tends to fall and an increased supply of a commodity becomes possible at a given market price.

Both demand and supply functions independently serve important functions. However, it is important to bring them together in an attempt to establish equilibrium. The concept of equilibrium, though analytical in nature, is quite simple in practice. It can be defined as a point of equality or agreement between buyers and sellers. Since both demand and supply quantities are shown in the scheduled forms these indicate mutual willingness of consumers and producers to purchase or sell respectively, varying quantities at varying prices. The schedules do not yet explain actual market price at which deals take place. This can be possible only when the quantities demanded and supplied are exactly equal at some uniform price. So long as this has not been achieved, some buyers or sellers are yet dissatisfied and may attempt to raise or lower the price. In this sense equilibrium is a point of complete satisfaction of the given behavior of buying and selling and hence an act of fulfillment of a given economic activity.

Let’s present and illustrate the establishment of equilibrium with the help of demand and supply functions in our earlier examples (in the sections given above). We begin with two equations:

q_d = 10 - 3P and q_s = 2P

By definition, demand and supply must be equal (q_d = q_s) for the condition of equilibrium.

q_d = 10 - 3P = q_s = 2 P or 10 - 3P = 2P

On solving this we find equilibrium price:

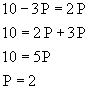
On substituting the value of price in demand and supply function we get,

qd = 10 - 3P qd = 10 - 3 (2) = 10 - 6 = 4

qs = 2P qs = 2 (2) = 4

Hence equilibrium price is 2, at which both quantity demanded and supplied are equal to 4. The algebraic proof of the equilibrium can be presented geometrically.

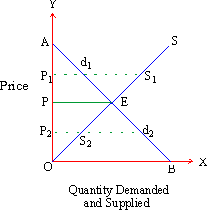
Figure 6

In the figure, AB and OS are the demand and supply curves respectively. The two curves intersect at point E which is an equilibrium point at which price P = 2 and quantity demanded and supplied are both equal (q = 4). At any other price higher than P such as P₁, the quantity supplied S₁ exceeds the quantity demanded d₁ (S₁ > d₁) and hence at this stage, some sellers remain dissatisfied. On the other hand at any lower price such as P₂, quantity demanded d₂ exceeds quantity supplied S₁ (d₂ > S₁) and this time some buyers remain dissatisfied. Therefore only at the point of intersection between demand and supply curves can equilibrium be attained. In other words, equilibrium price represents that price at which buyers are willing to buy the good and sellers are willing to sell it. This is the point of satisfaction for both the groups.

commodity: Vare

Consumer: forbruger

measure: måle

intersect: skære

assume zero value: Antage nulværdi

slope downward: hælde nedad

inverse: omvendt

shape: form

Ceteris Paribus: Alt andet lige

State: formulere

Marginal: Grænse-

Thus: Således

Contract: Trække sig sammen

restrain: Formindske

goods: Varer

However: Imidlertid

unit: enhed

range: række, interval

Equilibrium: ligevægt

increase: Forøge

Quantity: mængde